Shapes 4 4 9
Concrete blocks, also known as Concrete Masonry Units or CMUs, provide very durable structural and non-structural partitions. They are generally used as a backup wall that gets covered with a finish material or exposed in utilitarian spaces like mechanical rooms or basements. They can be painted to provide a cleaner finish, but they are also manufactured with different finishes that help improve the aesthetic quality over the traditional gray CMU.
Lamina shapes include 2D figures that can be drawn on a plane, e.g., circle, square, triangle, rectangle, trapezium, rhombus and parallelogram. Area of shapes such as circle, triangle, square, rectangle, parallelogram, etc. Are the region occupied by them in space. Polygon shape: A polygon is a two-dimensional shape that is formed by straight. Jan 04, 2018 Different Shapes Names in English! List of shapes with different types and useful example sentences. If you work in a business that requires the use of mathematics, for example then it would be very important that you are aware of the English names for shapes. However, this may not be the only reason that you need to learn this information.
Nominal vs. Actual Dimensions
As with brick, CMUs have actual dimensions and nominal dimensions. The nominal dimension of a CMU is the actual dimension plus the width of the mortar joint. Typical CMU mortar joints are 3/8'. The nominal dimension works within the 4' grid that other construction materials follow. The graphic below shows the difference between nominal and actual sizes.
Concrete Block (CMU) Sizes
Concrete Masonry Units (CMUs) are manufactured in a variety of sizes. They are identified by their depth – i.e. the thickness of the wall they create. For instance, a 6' CMU is nominally 6' deep while a 10' CMU is nominally 10' deep.
Concrete blocks also come in half-sizes, which are helpful in reducing the need to cut blocks in the field at corners or the end of walls. The architect should always attempt to design buildings using the nominal dimensions to the nearest half-block size to reduce waste and labor due to cut blocks.
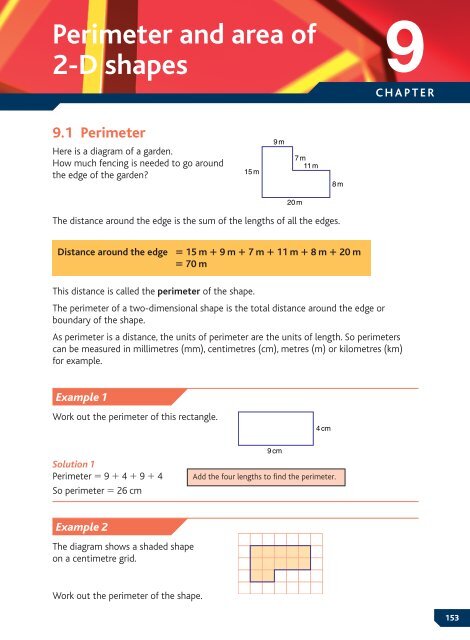
The following table provides concrete block sizes in both nominal and actual dimensions. While 4', 6', 8', 10', and 12' are the standard sizes, some manufacturers offer other sizes not listed below.
| CMU Size | Nominal Dimensions D x H x L | Actual Dimensions D x H x L |
|---|---|---|
| 4' CMU Full Block | 4' x 8' x 16' | 3 5/8' x 7 5/8' x 15 5/8' |
| 4' CMU Half-Block | 4' x 8' x 8' | 3 5/8' x 7 5/8' x 7 5/8' |
| 6' CMU Full Block | 6' x 8' x 16' | 5 5/8' x 7 5/8' x 15 5/8' |
| 6' CMU Half-Block | 6' x 8' x 8' | 5 5/8' x 7 5/8' x 7 5/8' |
| 8' CMU Full Block | 8' x 8' x 16' | 7 5/8' x 7 5/8' x 15 5/8' |
| 8' CMU Half-Block | 8' x 8' x 8' | 7 5/8' x 7 5/8' x 7 5/8' |
| 10' CMU Full Block | 10' x 8' x 16' | 9 5/8' x 7 5/8' x 15 5/8' |
| 10' CMU Half-Block | 10' x 8' x 8' | 9 5/8' x 7 5/8' x 7 5/8' |
| 12' CMU Full Block | 12' x 8' x 16' | 11 5/8' x 7 5/8' x 15 5/8' |
| 12' CMU Half-Block | 12' x 8' x 8' | 11 5/8' x 7 5/8' x 7 5/8' |
Concrete Masonry Unit (CMU) Specifications
CMU manufacturers must conform to ASTM standards when fabricating concrete blocks. The standards identify appropriate materials, compressive strength, dimensional tolerance, moisture absorption, and other performance characteristics.
Concrete brick is made from concrete similar to concrete block, but concrete bricks are fabricated in sizes that match traditional clay bricks (i.e. 2 2/3' tall).
The weight of concrete masonry units varies depending on the density of concrete mix used. There are three classifications of CMU: lightweight (less than 105 lb/ft3), medium weight (between 105 lb/ft3 and 125 lb/ft
The following table provides the ASTM standards that apply to concrete masonry units. ASTM C90 covers most conventional CMU construction and is the standard referenced by the ICC codes.
| ASTM Designtaion | Type of CMU |
|---|---|
| ATSM C55 | Concrete Brick |
| ASTM C73 | Calcium Silicate Face Brick |
| ASTM C90 | Load Bearing Concrete Masonry Units |
| ASTM C139 | CMUs for construction of Catch Basins and Manholes |
| ASTM C744 | Prefaced Concrete and Calcium Silicate Masonry Units |
| ASTM C936 | Solid Interlocking Concrete Paving Units |
| ASTM C1372 | Segmental Retaining Wall Units |
Concrete Masonry Unit (CMU) Shapes
There are an almost infinite number of CMU shapes available. The images below cover the most common shapes, but you should check with your local CMU manufacturer if you need special shapes or finishes.
Concrete Masonry Unit (CMU) Surface Finishes
As with shapes, there are a large number of finish options so you can make the utilitarian gray concrete block look much more aesthetically pleasing. Be sure to meet with your local CMU representative to learn about what types of finishes they offer and at what cost premium.
Colored CMU
Pigments can be added to both the concrete block as well as the mortar. The allows for the block and mortar to blend together or to have a sharp contrast. Keep in mind that there will be a natural variation in the color of the CMUs, even if they come from the same manufacturing batch. Therefore, it is important that the architect review at least 3 sample blocks that demonstrate the expected color variation.
If the color needs to be consistent or exact, you may consider painting the block and mortar. Painting is generally done on the interior, but can also be accomplished on exterior surfaces. Be sure to work with your CMU manufacturer and paint supplier to select the right paint for your situation.
Glazed CMU
Glazed CMU has a tile-like glazed finish made from a resin-based coating. The coating offers a wide variety of color as well as some faux material patterns. These surfaces may offer improved resistance to graffiti and chemicals. Glazed CMU is covered via ASTM C744, but the blocks still need to adhere to the ASTM C90 standard for load bearing CMU.
Split Faced CMU
Split faced CMU is manufactured by molding two units side-by-side and then mechanically splitting them apart after firing. This creates a very rough texture that some people think looks like stone, especially since the aggregates in the block are also split or exposed.
Ribbed or scored units (graphics above in the Shapes section) can also have a split faced finish to add more texture.
Soft-Split CMU
Soft-Split CMU is made with special molds that create a split-faced appearance instead of mechanically splitting the blocks. The effect is a bit less rough than split faced. In addition, the mold means that aggregates are not exposed.
Burnished or Polished CMU
Also referred to as Ground Face CMU, concrete blocks can be burnished or polished to expose the natural aggregates in the concrete mix. It is important to work with the manufacturer to select appealing aggregate and to test the polishing process to ensure you get the aesthetic you are looking for.
Sandblasted CMU
Sandblasting concrete masonry units also exposes the aggregate, but also blasts away some of the sand and cement to create a rougher appearance. Some people believe this creates a natural weathered look instead of the smoother look of burnished or polished CMU.
Raked CMU
Raked (or Striated) units have vertical rake marks introduced during the molding process. The rake marks are not as deep as the scored or ribbed blocks identified above in the Shapes section, but they do add an appealing texture to the block. You can also specify that scored or ribbed blocks have an additional raked pattern applied for more texture.
Area is the size of a surface!
It helps to imagine how much paint would cover the shape.
Area of Simple Shapes
There are special formulas for certain shapes:

Example: What is the area of this rectangle?
The formula is:
Area = w × h
w = width
h = height
The width is 5, and the height is 3, so we know w = 5 and h = 3:
Area = 5 × 3 = 15
Learn more at Area of Plane Shapes.
Area by Counting Squares
We can also put the shape on a grid and count the number of squares:
The rectangle has an area of 15
Example: When each square is 1 meter on a side, then the area is 15 m2 (15 square meters)
Square Meter vs Meter Square
The basic unit of area in the metric system is the square meter, which is a square that has 1 meter on each side:
1 square meter
Shapes 4 4 9 Images
Be careful to say 'square meters' not 'meters squared':
There are also 'square mm', 'square cm' etc, learn more at Metric Area.
Approximate Area by Counting Squares
Sometimes the squares don't match the shape exactly, but we can get an 'approximate' answer.
One way is:
- more than half a square counts as 1
- less than half a square counts as 0
Like this:
This pentagon has an area of approximately 17
Or we can count one square when the areas seem to add up.
Example: Here the area marked '4' seems equal to about 1 whole square (also for '8'):
This circle has an area of approximately 14
But using a formula (when possible) is best:
Example: The circle has a radius of 2.1 meters:
The formula is:
Area = π × r2
Where:
- π = the number pi (3.1416...)
- r = radius
The radius is 2.1m, so:
So the circle has an area of 13.85 square meters (to 2 decimal places)
Area of Difficult Shapes
We can sometimes break a shape up into two or more simpler shapes:
Example: What is the area of this Shape?
Let's break the area into two parts:
Part A is a square:
Part B is a triangle. Viewed sideways it has a base of 20m and a height of 14m.
So the total area is:
Area = Area of A + Area of B
Area = 400m2 + 140m2
Area = 540m2
Area by Adding Up Triangles
We can also break up a shape into triangles:
Then measure the base (b) and height (h) of each triangle:
Then calculate each area (using Area = ½b × h) and add them all up.
Area by Coordinates
Shapes 4 4 9 Kjv
When we know the coordinates of each corner point we can use the Area of Irregular Polygons method.
There is an Area of a Polygon by Drawing Tool that can help too.